The MIT School of Engineering’s mission is to educate the next generation of engineering leaders, to create new knowledge, and to serve society.
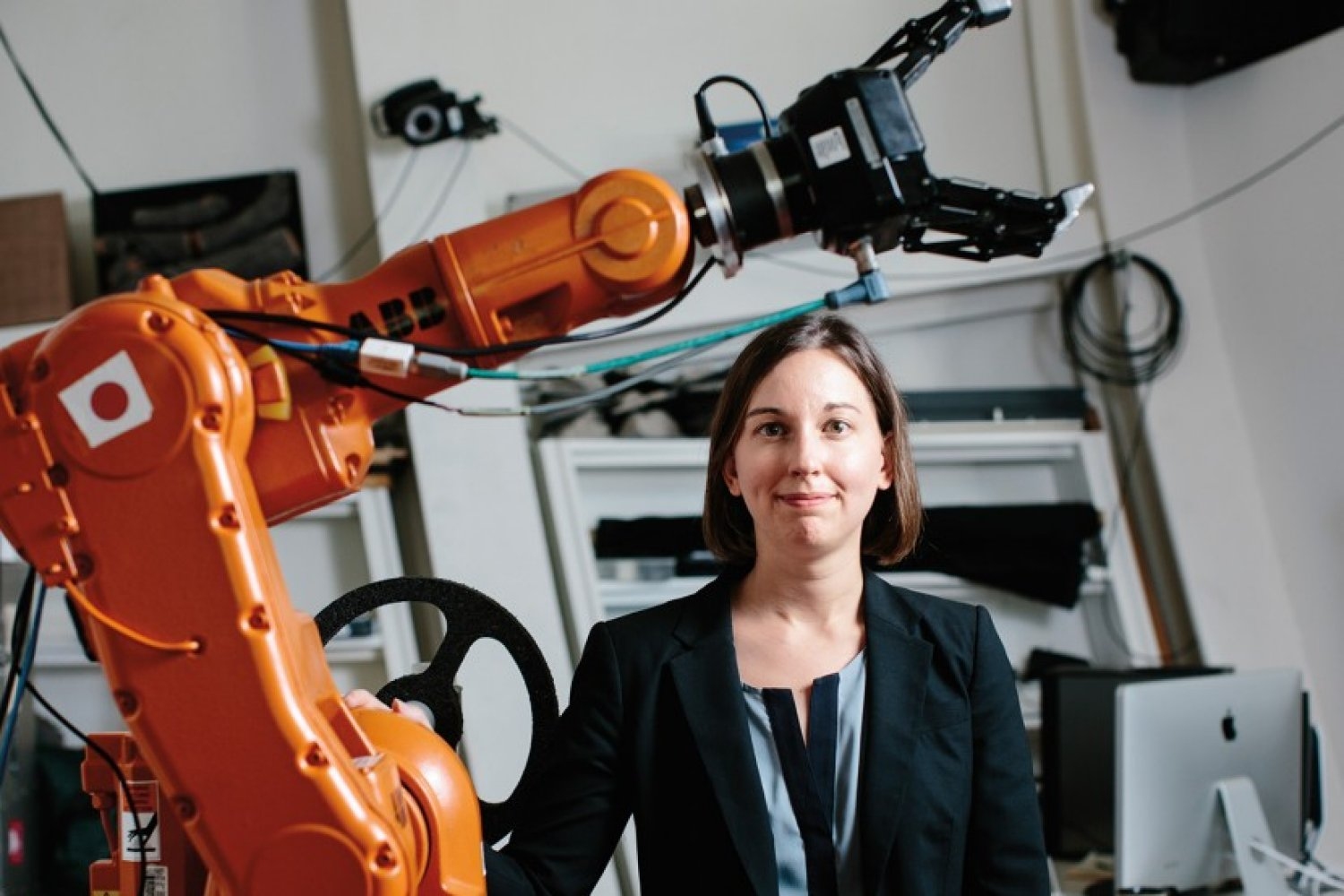
Julie Shah named head of the Department of Aeronautics a ...
Julie Shah ’04, SM ’06, PhD ’11, the H.N. Slater Professor in Aeronautics and Astronautics, has been named the new head of the Departmen ...

3 Questions: A shared vocabulary for how infectious dise ...
On April 18, the World Health Organization (WHO) released new guidance on airborne disease transmission that seeks to create a consensus ...
- Creating bespoke programming languages for efficient visual AI systems
- HPI-MIT design research collaboration creates powerful teams
- MIT conductive concrete consortium cements five-year research agreement with Japanese industry
- Exploring frontiers of mechanical engineering
- Three from MIT named 2024-25 Goldwater Scholars
Video Spotlight
Thriving Stars at MIT EECS
The Thriving Stars program in MIT’s Department of Electrical Engineering and Computer Science is on a mission to improve gender representation in electrical engineering and computer science.